Derivative Chart
Derivative Chart - 3.2 the derivative as a function; The slope of a constant value (like 3) is always 0. 3.4 derivatives as rates of change; Students, teachers, parents, and everyone can find solutions to their math problems instantly. You can also check your answers! Min, max, critical points, asymptotes, concavity, inflection, etc. D dx(f(x)g(x)) = f'(x)g(x) + f(x)g'(x) d d x ( f ( x) g ( x)) = f ′ ( x) g ( x) + f ( x) g ′ ( x) 4. [latex]\frac {d} {dx} (c)=0 [/latex] 2. It is available to download both as a pdf and word document. Those with hyperlinks have proofs. You should be able to use the table when other variables are used. [latex]\frac {d} {dx} (c)=0 [/latex] 2. [latex]\frac {d} {dx} (f (x)+g (x))= {f}^ {\prime } (x)+ {g}^ {\prime } (x) [/latex] 3. Web this pdf includes the derivatives of some basic functions, logarithmic and exponential functions. D dx(c) = 0 d d x ( c) = 0. The slope of a constant value (like 3) is always 0. The derivative rules are established using the definition. Another common interpretation is that the derivative gives us the slope of the line tangent to the function's graph at that point. 3.9 derivatives of exponential and logarithmic functions Students, teachers, parents, and everyone can find solutions to their math problems. Type in any function derivative to get the solution, steps and graph. The derivative tells us the slope of a function at any point. Gilbert strang & edwin “jed” herman. 3.2 the derivative as a function; The slope of a line like 2x is 2, or 3x is 3 etc. Web overview of the product rule for differentiation. You can also check your answers! Students, teachers, parents, and everyone can find solutions to their math problems instantly. D dx(f(x) + g(x)) = f'(x) + g'(x) d d x ( f ( x) + g ( x)) = f ′ ( x) + g ′ ( x) 3. 3.9 derivatives of. Learn all about derivatives and how to find them here. Web overview of the product rule for differentiation. Once we've established the derivative rules, we can use them to find derivatives more efficiently than using the definition each time. You can also check your answers! There are rules we can follow to find many derivatives. Web the big idea of differential calculus is the concept of the derivative, which essentially gives us the direction, or rate of change, of a function at any of its points. You should be able to use the table when other variables are used. Gilbert strang & edwin “jed” herman. Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. The slope of. Apart from these formulas, pdf also covered the derivatives of trigonometric functions and inverse trigonometric functions as well as rules of differentiation. Web this pdf includes the derivatives of some basic functions, logarithmic and exponential functions. There are rules we can follow to find many derivatives. Once we've established the derivative rules, we can use them to find derivatives more. [latex]\frac {d} {dx} (f (x)g (x))= {f}^ {\prime } (x)g (x)+f (x) {g}^ {\prime } (x) [/latex] 4. [latex]\frac {d} {dx} (f (x)+g (x))= {f}^ {\prime } (x)+ {g}^ {\prime } (x) [/latex] 3. The derivative tells us the slope of a function at any point. 3.9 derivatives of exponential and logarithmic functions Web the big idea of differential calculus. Free math lessons and math homework help from basic math. Derivative of the identity function. Check out this free printable derivatives formula chart i created for my ap calculus students to use as a reference. Gilbert strang & edwin “jed” herman. It is available to download both as a pdf and word document. 3.2 the derivative as a function; 3.5 derivatives of trigonometric functions; Free math lessons and math homework help from basic math to algebra, geometry and beyond. Another common interpretation is that the derivative gives us the slope of the line tangent to the function's graph at that point. Min, max, critical points, asymptotes, concavity, inflection, etc. [latex]\frac {d} {dx} (c)=0 [/latex] 2. Jenn, founder calcworkshop ® , 15+ years experience (licensed & certified teacher) that’s what finding derivatives using a table of values or graphs is all about, and it’s relatively straightforward! 3.9 derivatives of exponential and logarithmic functions The derivative tells us the slope of a function at any point. You should be able to use the table when other variables are used. [latex]\frac {d} {dx} (f (x)+g (x))= {f}^ {\prime } (x)+ {g}^ {\prime } (x) [/latex] 3. Free math lessons and math homework help from basic math. Web this pdf includes the derivatives of some basic functions, logarithmic and exponential functions. Derivative of the identity function. D dx(c) = 0 d d x ( c) = 0. In each case, use the table of derivatives to write down. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Web visualizing a derivative | desmos. Web shares of tesla inc. D dx(f(x)g(x)) = f'(x)g(x) + f(x)g'(x) d d x ( f ( x) g ( x)) = f ′ ( x) g ( x) + f ( x) g ′ ( x) 4. The table below shows you how to differentiate and integrate 18 of the most common functions.
Calculus Derivative Table
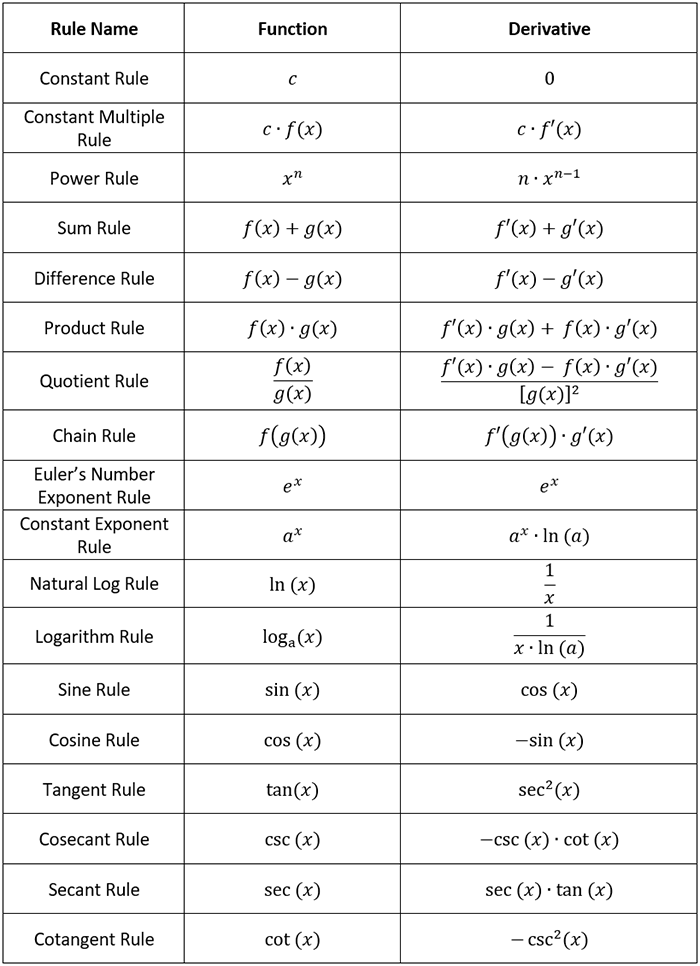
Derivative Table
Free Printable Derivatives Formula Chart (PDF) Math = Love
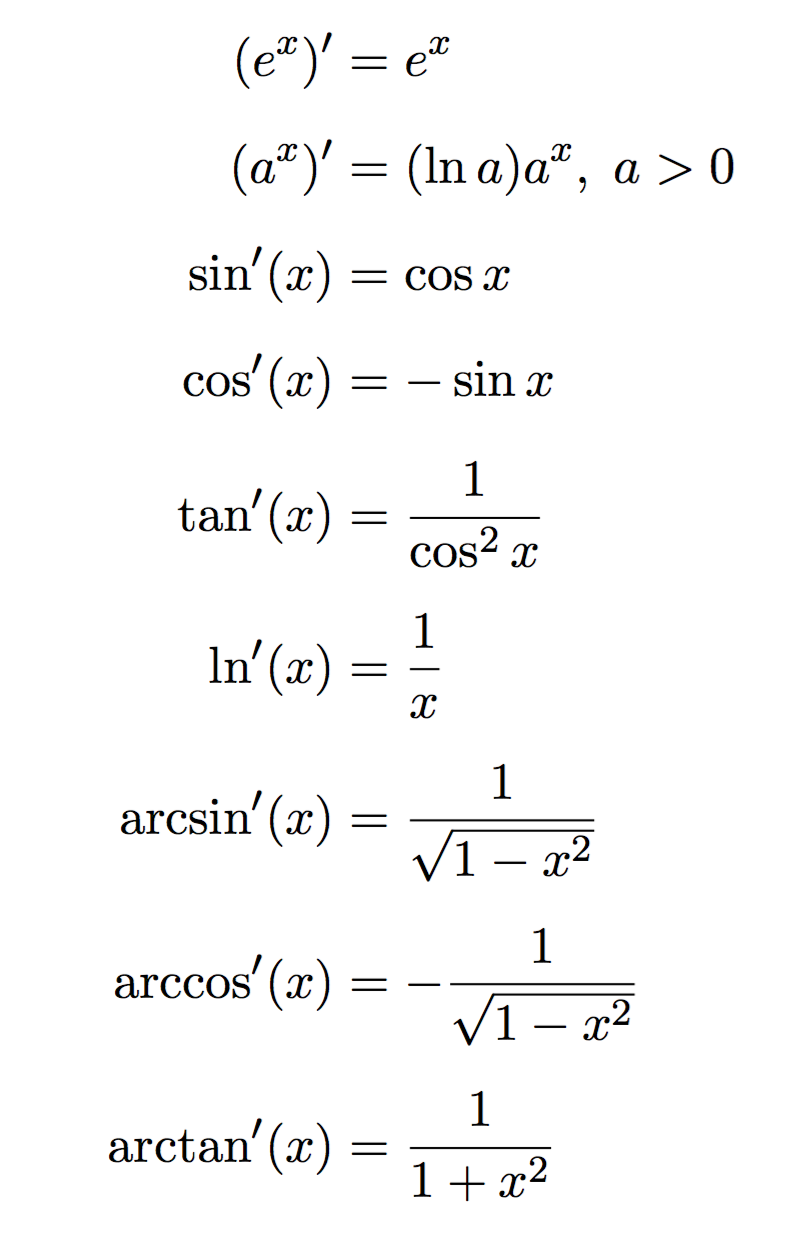
Basic derivatives
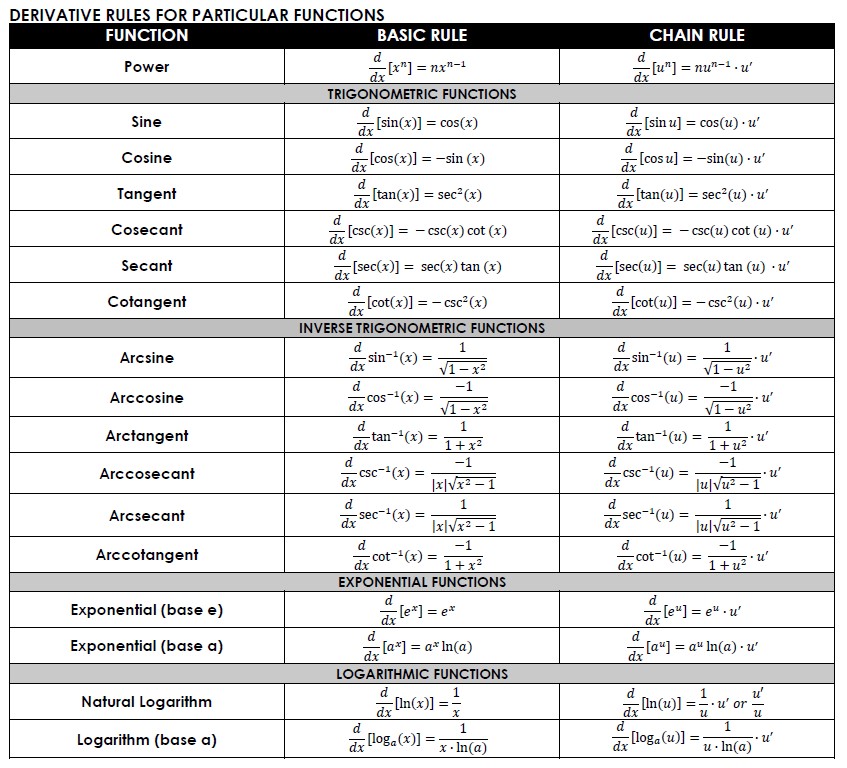
Free Printable Derivatives Formula Chart (PDF) Math = Love
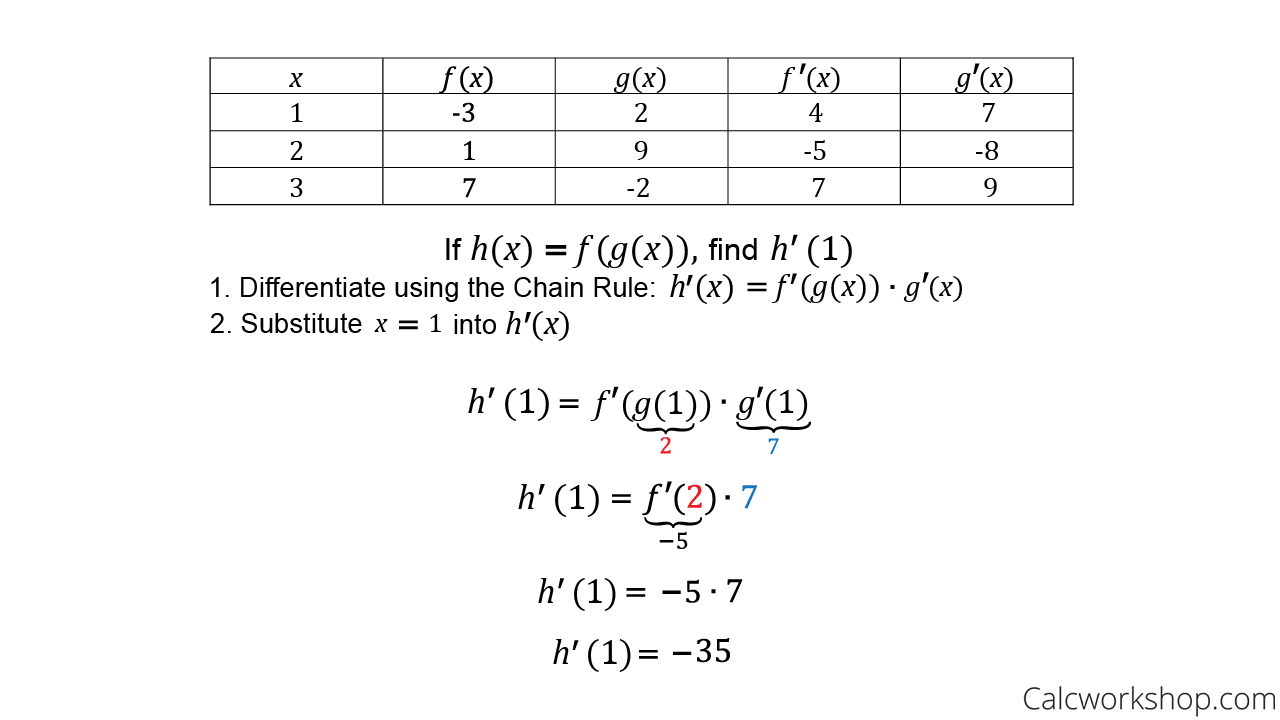
Derivatives Using Charts (Fully Explained w/ Examples!)

Higher Order Derivatives
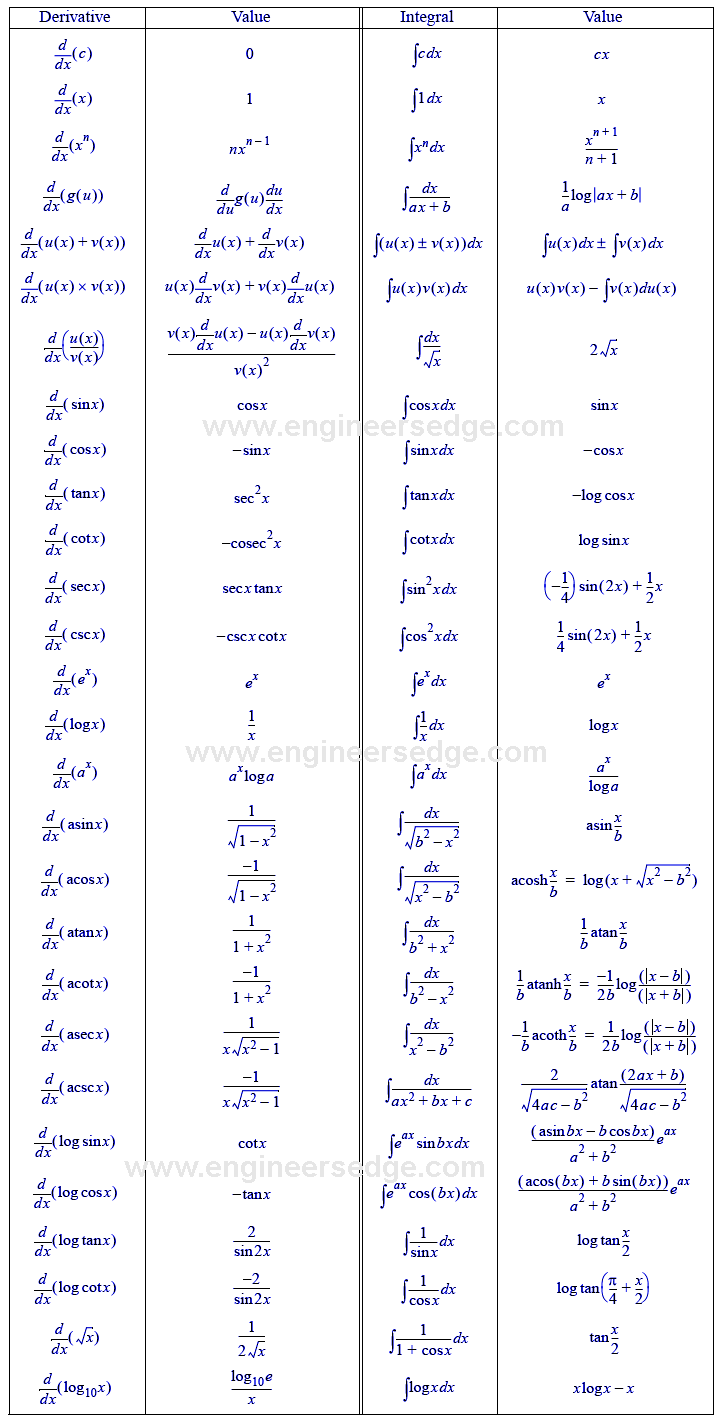
Integrals and Derivatives of Function Chart

Derivative Rules Power, Product and Sum rules Math Original
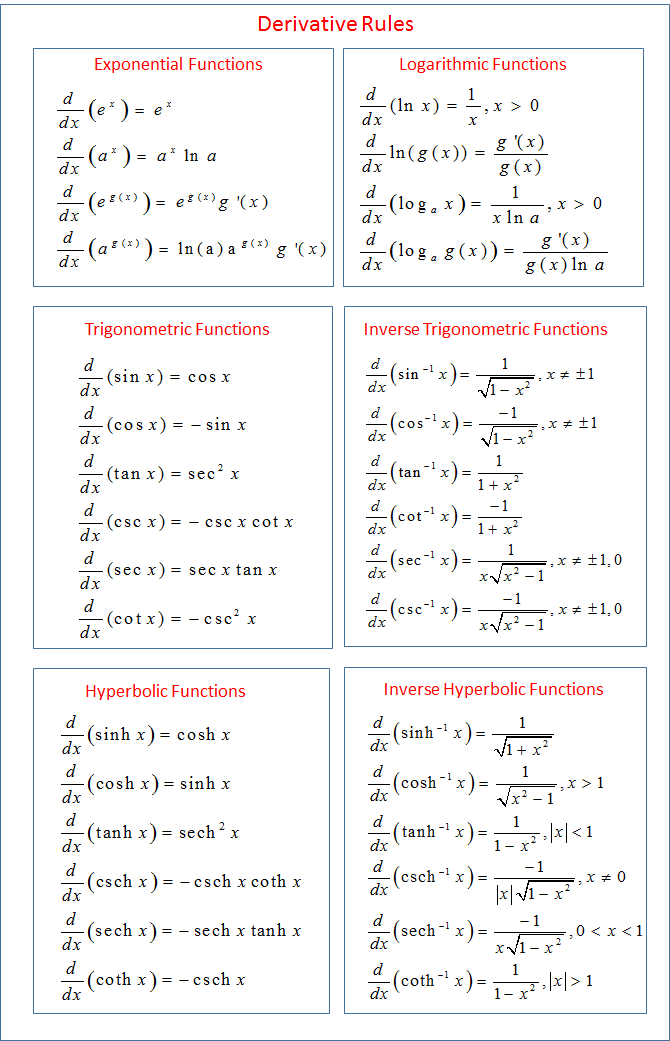
Calculus Derivative Rules (video lessons, examples, solutions)
Type In Any Function Derivative To Get The Solution, Steps And Graph.
Another Common Interpretation Is That The Derivative Gives Us The Slope Of The Line Tangent To The Function's Graph At That Point.
Web The Derivative Of A Function Describes The Function's Instantaneous Rate Of Change At A Certain Point.
There Are Rules We Can Follow To Find Many Derivatives.
Related Post: