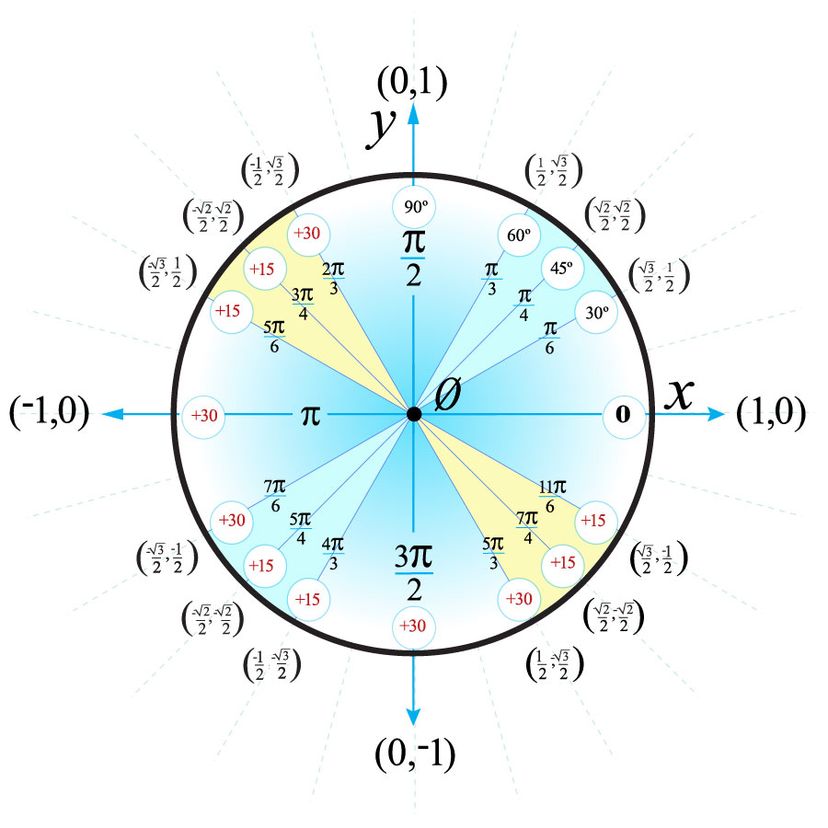
Unit Circle Drawing
Unit Circle Drawing - The unit circle is generally represented in the cartesian coordinate plane. So why is it so useful? In a circle or on a graph. Web what is the unit circle and why is it important in trigonometry? Web what is unit circle? Imagine that you stop before the circle is completed. It describes all the negatives and positive angles in the circle. Split it down the middle. Recall that a unit circle is a circle centered at the origin with radius 1, as shown in figure 2. How to memorize the unit circle. Sine, cosine and tangent (often shortened to sin, cos and tan) are each a ratio of sides of a right angled triangle: For a given angle θ each ratio stays the same. Split it down the middle. We can see things in their simplest form. The unit circle is generally represented in the cartesian coordinate plane. In this video i show you how. How do we associate an arc on the unit circle with a closed interval of real numbers? Sine, cosine and tangent (often shortened to sin, cos and tan) are each a ratio of sides of a right angled triangle: The angle (in radians) that t t intercepts forms an arc of length s.. Web you don't need to memorize the entire unit circle to be able to draw it from scratch. For a given angle θ each ratio stays the same. Let's get an intuition of the unit circle by using the interactive below. Web an interactive for exploring the coordinates and angles of the unit circle, as well as finding the patterns. The portion that you drew is referred to as an arc. In a circle or on a graph. Web an interactive for exploring the coordinates and angles of the unit circle, as well as finding the patterns among both. Web you don't need to memorize the entire unit circle to be able to draw it from scratch. In this video. No matter how big or small the triangle is. The angle (in radians) that \(t\) intercepts forms an arc of length \(s\). Frequently, especially in trigonometry, the unit circle is the circle of radius 1 centered at the origin (0, 0) in the cartesian coordinate system in the euclidean plane. Being so simple, it is a great way to learn. Web an interactive for exploring the coordinates and angles of the unit circle, as well as finding the patterns among both. Imagine that you stop before the circle is completed. The unit circle is simple, it's a circle with a radius of 1. How do we associate an arc on the unit circle with a closed interval of real numbers?. This line is at right angles to the hypotenuse at the unit circle and touches the unit circle only at that point (the tangent point). Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Web using the unit circle diagram, draw a line “tangent” to the unit circle where the hypotenuse contacts the unit circle. Generalizing. No matter how big or small the triangle is. Web everything you see in the unit circle is created from just three right triangles, that we will draw in the first quadrant, and the other 12 angles are found by following a simple pattern! A unit circle is a circle with a radius measuring 1 unit. How to memorize the. We have already defined the trigonometric functions in terms of right triangles. So why is it so useful? Recall that a unit circle is a circle centered at the origin with radius 1, as shown in figure 2. Draw an equilateral triangle with side length 2. It describes all the negatives and positive angles in the circle. Web the unit circle is a circle with a radius of 1. How do we associate an arc on the unit circle with a closed interval of real numbers? Draw an equilateral triangle with side length 2. What is meant by “wrapping the number line around the unit circle?” how is this used to identify real numbers as the lengths. So why is it so useful? Split it down the middle. Web to find another unit, think of the process of drawing a circle. Web what is the unit circle and why is it important in trigonometry? The unit circle is simple, it's a circle with a radius of 1. Web everything you see in the unit circle is created from just three right triangles, that we will draw in the first quadrant, and the other 12 angles are found by following a simple pattern! Web you don't need to memorize the entire unit circle to be able to draw it from scratch. Web finding trigonometric functions using the unit circle. For a given angle θ each ratio stays the same. In a circle or on a graph. Being so simple, it is a great way to learn and talk about lengths and angles. What is the equation for the unit circle? 3π/2, and 2π (in radians) respectively. Web a unit circle diagram is a platform used to explain trigonometry. Web a unit circle is divided into four quadrants making an angle of 90°, 180°, 270°, and 360° (in degrees) or π/2, π. No matter how big or small the triangle is.
Unit Circle Quick Lesson Printable PDF Chart · Matter of Math
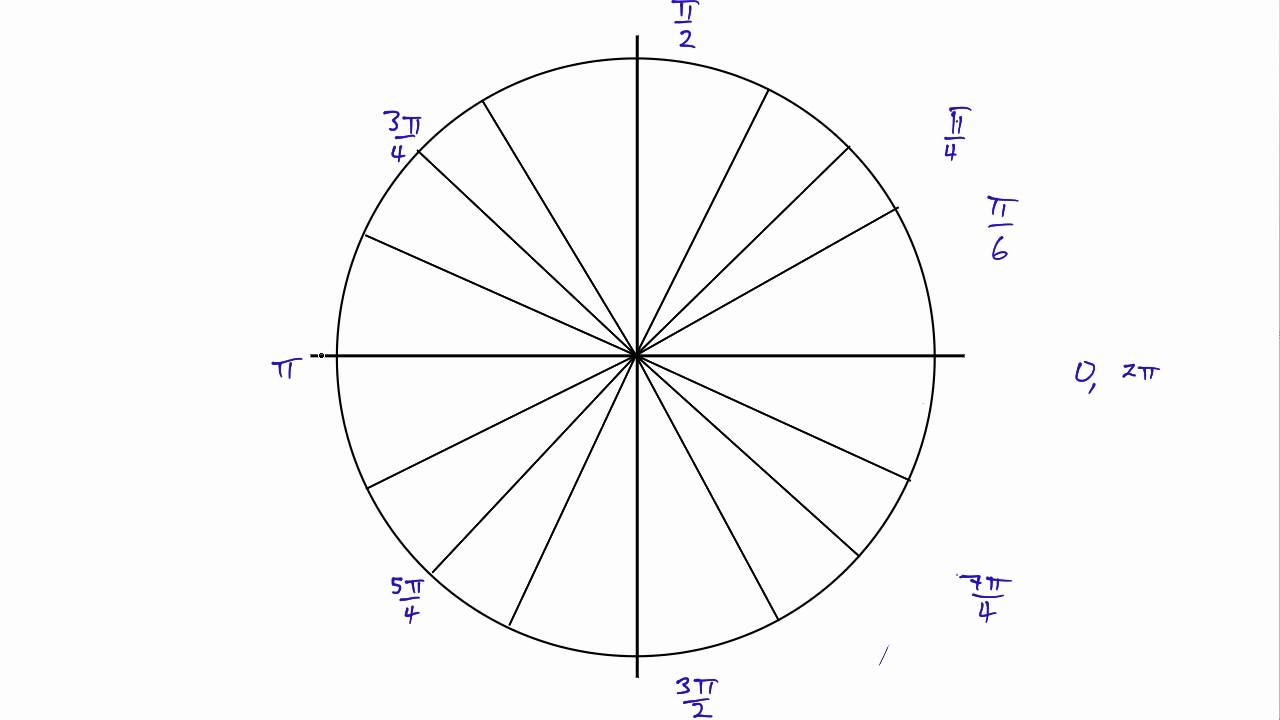
Drawing a Unit Circle YouTube
How to Use the Unit Circle in Trigonometry HowStuffWorks
Unit Circle Labeled At Special Angles ClipArt ETC

Unit Circle Quick Lesson Downloadable PDF Chart · Matter of Math
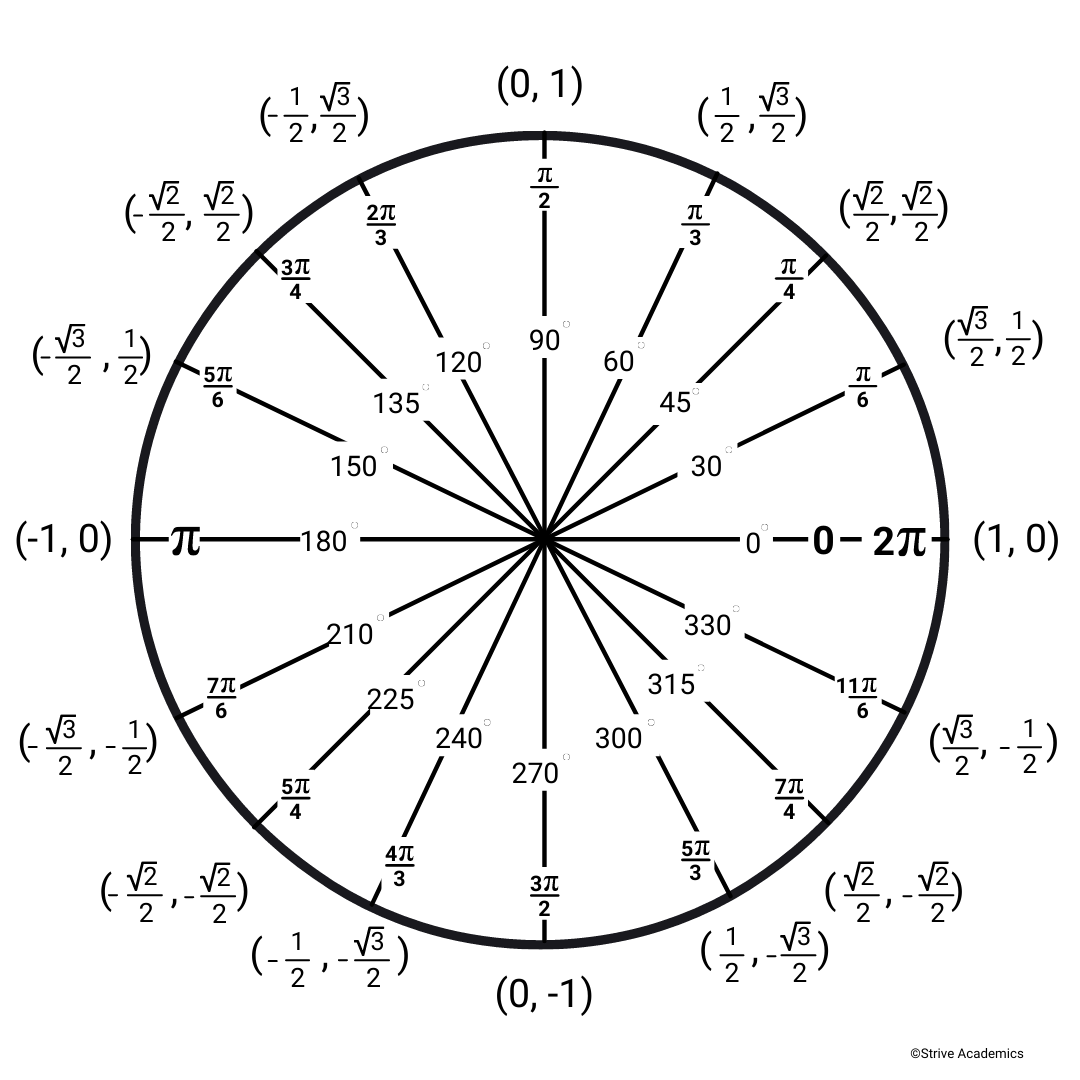
Unit Circle Diagrams Classful

Unit Circle Unit circle trigonometry, Math projects, Teaching math

Learn How to Draw a Unit Circle Without Memorizing It DevinCrystie
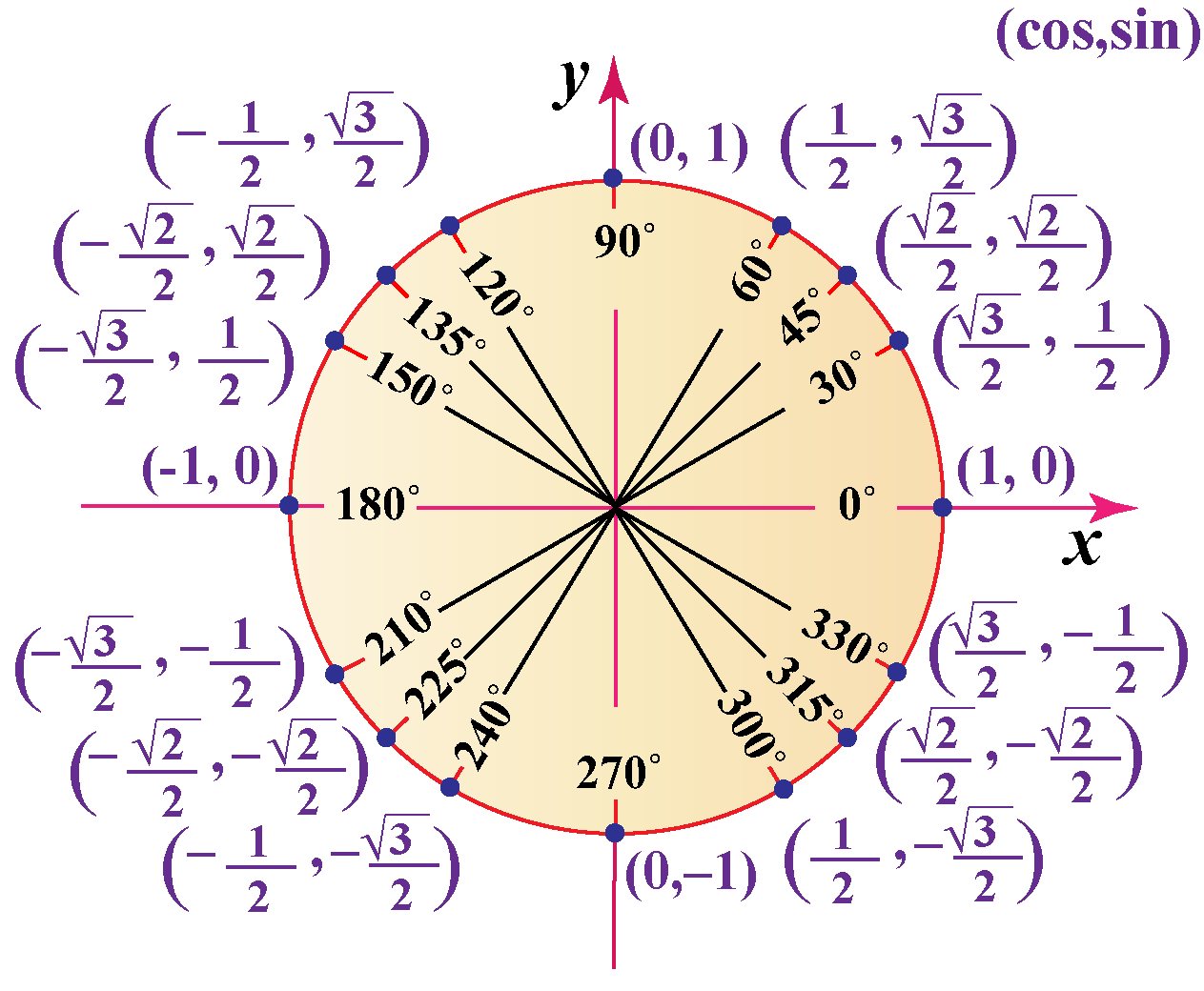
Unit circle Solved Examples Geometry Cuemath
Unit Circle Labeled With Special Angles And Values ClipArt ETC
Draw An Equilateral Triangle With Side Length 2.
Web The Unit Circle Is A Circle With A Radius Of 1.
It Describes All The Negatives And Positive Angles In The Circle.
The Angle (In Radians) That \(T\) Intercepts Forms An Arc Of Length \(S\).
Related Post: