Special Factoring Patterns
Special Factoring Patterns - If you learn to recognize these kinds of. The difference of squares pattern. Web the other two special factoring formulas you'll need to memorize are very similar to one another; Web in this article, we'll learn how to factor perfect square trinomials using special patterns. Web factoring using structure involves recognizing patterns in expressions, like the difference of squares or perfect square trinomials, and using these patterns to break down complex. Factor sums and differences of cubes. Web 5.6 special factoring formulas. Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. If you learn to recognize. Use foil and multiply (a+b)(a+b). Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. Web here are the special factor patterns you should be able to recognize. Web sal is using the pattern created by squaring a binomial. In this article, you will. Web 5.6 special factoring formulas. Web factoring with special forms is a process of using identities to help with different factoring problems. They're the formulas for factoring the sums and the differences of cubes. This reverses the process of squaring a binomial, so you'll want to understand that. When you learn to factor quadratics, there are three other formulas that. Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. Web by the end of this section, you will be able to: (a+b)^2 = a^2 + 2ab + b^2 here's where the 2 comes from. Web factoring using structure involves recognizing patterns in expressions, like the difference of squares or perfect square trinomials,. Use foil and multiply (a+b)(a+b). Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. The difference of squares pattern. When you learn to factor quadratics, there are three other formulas that they usually introduce at the same time. Web some interesting patterns arise when you are working with cubed quantities within polynomials. If you learn to recognize. Web factoring with special forms is a process of using identities to help with different factoring problems. Use foil and multiply (a+b)(a+b). The difference of squares pattern. Web by the end of this section, you will be able to: Web by the end of this section, you will be able to: Web learning to recognize a few common polynomial types will lessen the amount of time it takes to factor them. (a+b)^2 = a^2 + 2ab + b^2 here's where the 2 comes from. Web factorization goes the other way: This reverses the process of squaring a binomial, so. Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. If you learn to recognize. (a+b)^2 = a^2 + 2ab + b^2 here's where the 2 comes from. Factorize x 2 − 9 = 0. If you learn to recognize these kinds of. Use foil and multiply (a+b)(a+b). In this article, you will. Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. Web the other two special factoring formulas you'll need to memorize are very similar to one another; Web sal is using the pattern created by squaring a binomial. Memorize the formulas, because in some cases, it's very hard to generate them without wasting a lot. Web factoring with special forms is a process of using identities to help with different factoring problems. Factorize x 2 − 9 = 0. Web by the end of this section, you will be able to: If you learn to recognize these kinds. Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. When we learned how to multiply polynomials, we learned how to quickly multiply commonly occurring scenarios using special products formulas. Memorize the formulas, because in some cases, it's very hard to generate them without wasting a lot. The first is the difference of. (a+b)^2 = a^2 + 2ab + b^2 here's where the 2 comes from. If you learn to recognize. If the first and last terms of a trinomial are squares, try. Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. Web the formula used for factoring difference of squares is: Web by the end of this section, you will be able to: What you will learn in this lesson. Web we have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. When you learn to factor quadratics, there are three other formulas that they usually introduce at the same time. The perfect square trinomial pattern. Knowing the characteristic patterns of special products,. Factoring by difference of squares. The difference of squares pattern. Web here are the special factor patterns you should be able to recognize. This reverses the process of squaring a binomial, so you'll want to understand that. Specifically, there are two more special cases to consider: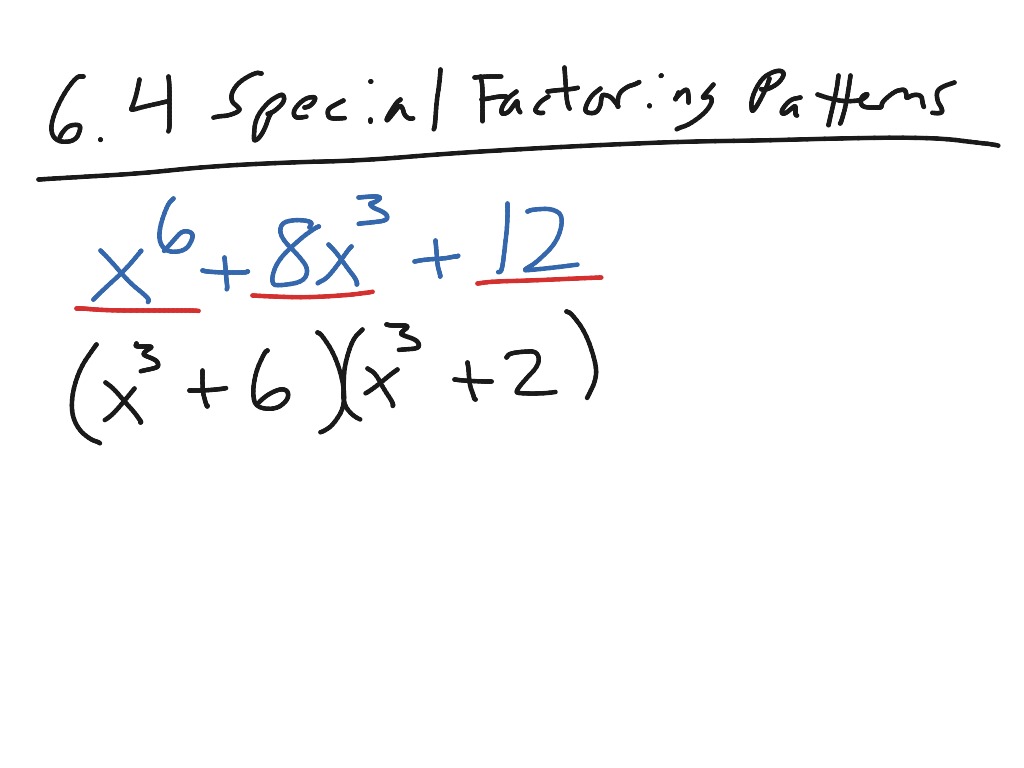
6.4 Special Factoring Patterns Math, Factoring Polynomials ShowMe

IM3Special Factoring Patterns YouTube

Ch.11.6 Special Factoring Patterns

Algebra 2 Unit 3 Day 5 Factoring Special Patterns and Grouping

factoring special patterns YouTube

Factoring Formulas in Algebra What Are Factoring Formulas?
Factoring INB Pages Mrs. E Teaches Math

Special factoring patterns YouTube
Factoring INB Pages Mrs. E Teaches Math

Factoring Special Patterns YouTube
When We Learned How To Multiply Polynomials, We Learned How To Quickly Multiply Commonly Occurring Scenarios Using Special Products Formulas.
Web The Difference Of Squares Is A Special Factoring Case Where The Quadratic Equation Is Of The Form A 2 −B 2.
Memorize The Formulas, Because In Some Cases, It's Very Hard To Generate Them Without Wasting A Lot.
Web We Have Seen That Some Binomials And Trinomials Result From Special Products—Squaring Binomials And Multiplying Conjugates.
Related Post: